Many of the students do not have given images about the math classroom. They feel it quite dull and boring. It is one of the most uninteresting subjects which give a tough time to many of the students. The main reason is that they are highly unable to grab mathematical concepts. Some of the times, it may be due to missing the previous. Students can easily access the slope calculator to find the slope of a line. It brings amazing facilitation to their life.
Slope and its Practical Application:
In mathematics, a slope can be regarded as a gradient that helps in the determination of line steepness. Besides this, slope also helps much in determining the direction of the line. The absolute value is of keen significance as it helps in the measurement of line steepness. For a steeper line, the value of the slope would be greater. The graph line contains certain steepness that must be measured.
Slope finds its practical application in architecture work where buildings, roads, etc. have to be constructed. The steepness of the area must be known for making a rational decision. It would avoid upcoming issues in the future. A slope calculator can provide as many outcomes as you like. So, you can enjoy having limitless calculations on it.
The interest in mathematics is not possible to develop until you explain the practical application to others. Students always look for practical applications that how and where they can apply the concept. The slope calculator has made it much easier to determine the slope of the graph line with accuracy.
Determination of Line Direction:
With the slope calculator, you can enjoy determining the direction of the line. The outcome obtained from the calculation is a marvelous source for indicating the line direction. When the value of the slope is found to be more than zero, then it represents the line is in an upward direction. It is indicative of an increasing line. When the value of the slope is determined to be less than zero, then the line is decreasing and has its direction from left to right.
It is indicative of a line in a downward direction. However, when the slope calculator reflects the slope value’s outcome to be equal to zero, it depicts a constant slope. In such a case, the line is in the horizontal direction. The undefined slop represents a vertical line.
Determination of Coordinates:
The graph line consists of coordinates at it’s both axis. No matter either it is an increasing or decreasing line, it contains both coordinates with some specific values. The coordinates are represented by x and y.
Here, x coordinate represents value for the x-axis, and y-coordinate represents value for the y-axis. The initial and final value of the x-coordinate is represented by x1 and x2, respectively. Similarly, the initial and final values of y-coordinate are represented by y1 and y2, respectively.
Slope Formula:
All the calculations are made super easy due to the slope formula. The data is allowed to process in the slope formula to get the results. The slope calculator determines the slope of the line on the basis of the slope formula. It is given as below:
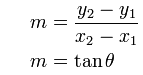
Where; m represents slope while θ is the angle of incline. The difference of final values of both coordinates from its initial value gives the nominator and denominator. The change in y-coordinate is kept as nominator, while the change in values of x coordinator is kept as x-coordinate. The slope calculator intends to determine the steepness of the line in the wink of an eye. The students must be highly focused and attentive to gain the concept to the optimum. Questioning and practice are necessary to master the skills.
In a Nutshell
The chances of error arise with manual calculations, and hence they must cross verify their results with the slope calculator. It would help them in rectifying the mistake, if any, at its earlier. Slope calculation starts when the line draw is accurate and visible. Now, take the ruler to determine the coordinates on it. After successfully determining both coordinators’ values, write down the values on the graph line with the use of a pen or pencil. Take the difference of both and then allow these to be divided for giving the slope value. It is the resultant value that provides an idea for steepness and lien direction.



